Length contraction and Special Relativity

The following thought experiment is a useful introduction to the idea of length contraction predicted by the special theory of relativity.

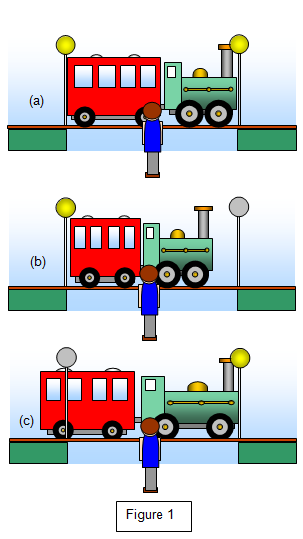
Think of a train and a bridge. At either end of the bridge there is a light and the light is controlled by a switch that operates between the track and the train. When the front of the train reaches the far side of the bridge the switch closes and the light there comes on. When the back of the train reaches the near side of the bridge the switch closes and so that light switches on.

An observer views the train from the centre of a path beneath the bridge.



If the train is the same length as the bridge the two lights come on together (Figure 1(a)).







If the train is shorter than the bridge the light on the near side of the bridge comes on before the far side one (Figure 1(b)).







If the train is longer than the bridge the light on the far side of the bridge comes on before the nearside one (Figure 1(c)).

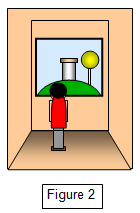
Now think what an observer inside the train sees. Imagine that they are travelling in a train that, according to an outside observer, is the same length as the distance between the two lights. Since they are moving towards the light on the far side of the bridge and away from the one on the near side of the bridge they see the far side light come on first (Figure 2) (they are moving away from the light coming from the near side). They therefore deduce that the train is longer than the bridge because it is similar to the result in Figure 1(c). This means that they think that the train is longer than the observer on the bank.

As predicted by special relativity lengths observed from the stationary reference frame look shorter than ones observed from within a reference frame moving relative to it.

Of course the time difference between the observer in the train receiving the light beams from the two lamps is insignificant at low speeds but this becomes larger as the speed of the train approaches that of light.