Lenses and waves

Lenses affect the light that passes through them making an image of the object from which the light waves come. Sometimes this image is real (you can form it on a surface such as a piece of paper) and sometimes virtual (it cannot be formed on a surface)

Curvature and the sign convention

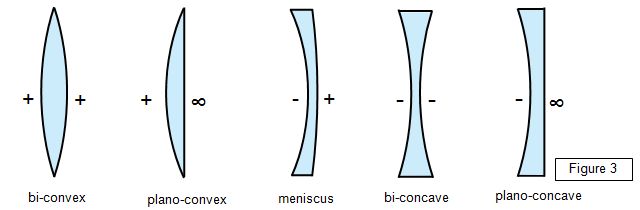
The radius of curvature (R) of a surface is taken as a positive number if it increases the curvature of the waves – i.e. converges the waves and negative if it decreases the curvature of the ways – i.e. diverges the waves.

A surface that converges a wavefront is taken a positive e.g. a convex lens

A surface that diverges a wavefront is taken as negative e.g. a concave lens

The curvature of the surface, or wave, is defined as 1/radius of that surface or wave. A surface with a small radius of curvature therefore has a large curvature and that with a large radius of curvature a small curvature.



Some examples of the radii of curvature of the surfaces of lenses are shown in Figure 3.

Waves and curved surfaces

When light waves fall on a curved surface that surface changes the curvature of the wave. Lenses are made with at least one curved surface (convex or concave) and so lenses simply change the curvature of the waves that fall on them.

Therefore:

Effect of a lens

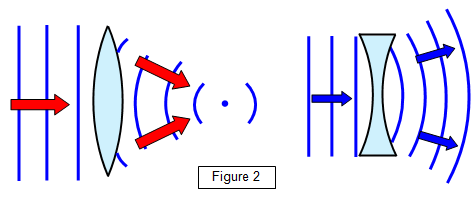
A lens adds curvature to a wavefront. As the waves enter the lens their curvature is changed. In the example below they are bent from a plane wave to form a converging wave in the lens. This wave is further converged when it leaves then lens.

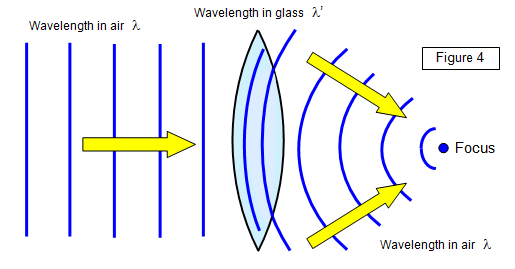
You can follow this change of curvature as the waves meet the lens and then pass through it, emerging at the other side.

As the wave hits the lens it is the centre of the wave that meets the glass first and so this part of the wave is slowed down first (light waves move slower in glass than they do in air). This means that the outer portions of the wave 'catch up' so increasing the curvature to form a converging beam.

As the wave leaves the lens the outer portions move into the air first and so speed up first. This means that the outer portions move off more rapidly first and so the curvature of the wave is further increased so converging the light more strongly.

The overall action of the convex lens is therefore to converge light waves to a focus.

Looking at the diagram you can see that waves with no curvature have been converged to a point a distance f (the focal length) from the lens. This means that they have been given a curvature 1/f. The shape of the incident waves have no effect on how much the lens changes their curvature and so we can say that a lens increases the curvature of waves that pass through it by a constant amount 1/f.

(Remember that 1/f will be positive for a convex lens and negative for a concave lens)

The example has been done with a plane wave but the same would occur with a spherical wave spreading outwards from a point object.

Wavefronts

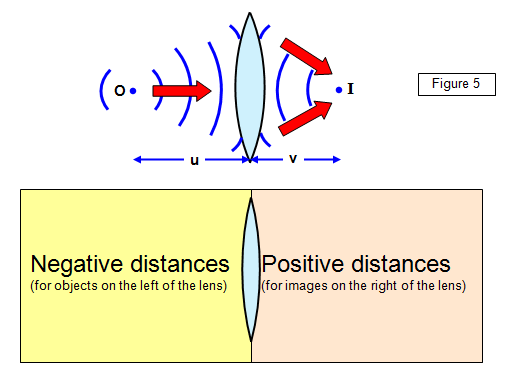
Wavefronts spreading outwards from a point source are taken as having a negative curvature and so the distance from the lens to the object is negative

Wavefronts that converge to an image are taken as having a positive curvature and so the distance from the lens to the image is positive.

Lens equation

The curvature of the waves spreading outwards towards a lens from an object distance u from the lens have a curvature 1/u and those converging to a point image on the other side of the lens have a curvature 1/v. The lens adds a constant curvature (1/f) to the wave and so the equation relating the object distance (u) image distance (v) and the focal length (f) is:

using the above sign convention called the Cartesian sign convention

This can be shown in the following diagram.