Absorption of X-rays

When X-rays pass through matter such as a human body they will lose energy in one or more of the following ways:

(a) Scattering – the energy of the X ray photon is not sufficient to cause electron emission from the atom (see Compton scattering) – 1 to 30 keV

(b) The photoelectric effect - an X- ray photon transfers all its energy to an electron which then escapes from the atom – 1 to 100 keV

(c) Compton scattering - an X-ray photon collides with a loosely-bound outer electron. At the collision the electron gains some energy and a scattered X-ray photon is produced travelling in a different direction from the incident photon and with a lower energy – 0.5 to 5 MeV

(d) Pair production - an X-ray photon with an energy greater than 1.02 MeV enters the intense electric field at the nucleus. It may be converted into particles, a positron and an electron. These two particles usually annihilate each other producing two photons, each of energy 511 keV – above 5 MeV.

In a vacuum the intensity (I) of the X ray beam will fall off as the inverse of the square of the distance (d) from the source.
I = I_o/d²
In a material where one or more of the absorption processes mentioned above occur the intensity of the beam falls of by a constant fraction dI as the beam passes though unit distance of the material.

In other words: -dI/I = mdx where m is a constant for the material.

The constant (m) is known as the total linear attenuation coefficient and this depends on both the medium and the energy of the X rays.

Half value thickness
This is the thickness of the material which is needed to reduce the intensity (I) of the X ray beam to one half the intensity of the incident radiation (I_o).

Since : I = I_oe^-mx if I/I_o = ½ then e^-mx = ½ and so





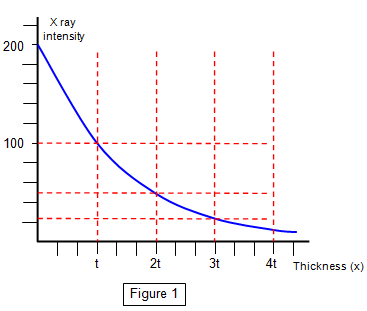
The graph shown in Figure 1 is for an X beam of one energy - monoenergetic. In this case the half value thickness of the material is always the same no matter how much the original intensity has been reduced. If the beam has a spread of energies then the further through the material the X ray beam passes the longer the half value thickness becomes.


This is because the lower energy X rays in the beam are absorbed more easily than the higher energy ones.
This is shown in Figure 2. The X ray beam contains a greater proportion of higher energy X rays the further into the material it penetrates. This is said to be a 'hardening' of the beam.



Therefore as the beam goes deeper into the material the 'average' energy of the X rays in the beam increases and so a greater thickness of absorber is needed to further reduce the beam intensity by a half.

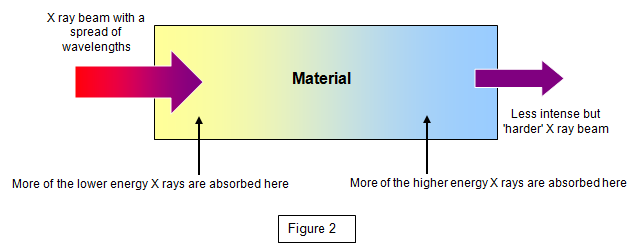
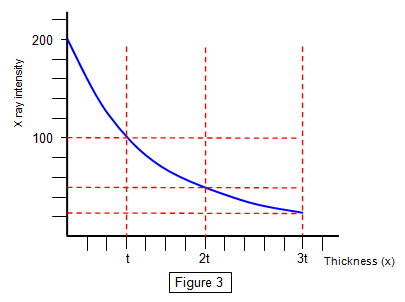
Figure 3 shows the graph for a beam of X rays with a spread of energy. It shows that the absorption curve flattens as the beam penetrates further into the material and the half value thickness also increases.

The equivalent energy of an X ray beam is shown by its half value thickness in a particular material. For example X rays from an X ray tube with a peak anode voltage of 80 kV have a half value thickness of about 3 mm of aluminium. However the half value thickness for a 28 keV monoenergetic beam of X rays is 3 mm of aluminium and so the equivalent energy of the 80 kV X ray beam is 28 keV.

The quality of the beam is determined by the spread of X ray energies across the spectrum

(See: 16-19/Atomic physics/X rays/Text/X ray spectra)