Vectors in equilibrium

For an object to be in equilibrium the following two conditions must be satisfied.



It follows from the first condition that if three non-parallel vectors (such as forces) act in an object that is in equilibrium then the lines of action of these three vectors must pass through one point.

The vectors can be represented in magnitude and direction by the three sides of a triangle – the vectors must be drawn so that their direction follows round the figure.

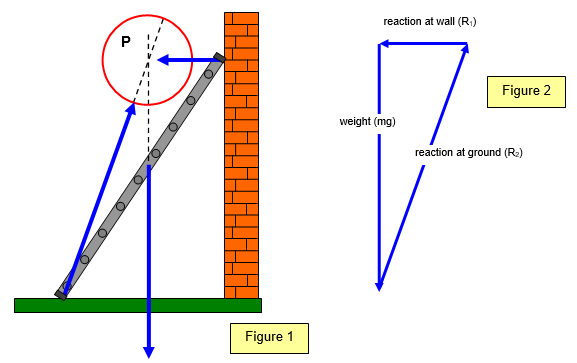
Vectors in equilibrium Ladder

A good example of the case of vectors in equilibrium is a ladder resting on rough ground and leaning against a smooth wall - see Figure 1.
Notice that the three forces involved are:
(a) the weight of the ladder
(b) the reaction at the wall (R1) – at right angles because the wall is smooth
(b) the reaction at the ground (R2) - not at right angles as the ground is rough
and that they all pass through the same point P.

The vector diagram for the three forces involved is shown in Figure 2.




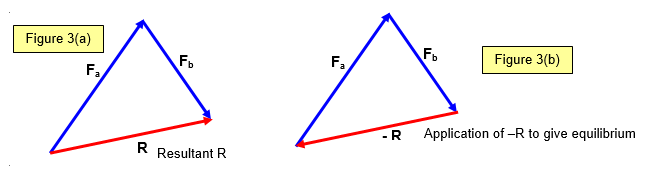
If two forces (Fa and Fb) act on an object (Figure 3(a)) then if the reverse of the resultant (R) is applied to the object as well the system will be in equilibrium (Figure 3(b)).


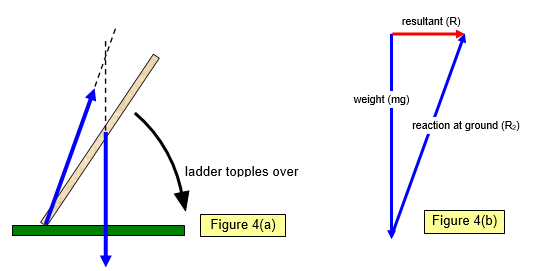
Now returning to the case of the ladder.
If the wall is suddenly taken away there are only two forces acting on the ladder – its weight and the reaction at the ground. These two would give a resultant R and the ladder would topple over to the right (Figures 4(a) and (b). Applying the reverse of R (putting back the wall) and making sure that all three forces passed through the same point would hold the ladder in place again.



Two versions of the ladder problem are now considered. The first using only the resolution of vectors and the second using moments and the resolution of vectors.