
When we
think about the speed of an object it is important to understand that there are various ways of
expressing this.
(a) Average value of a quantity
Imagine that you go on a
journey of 188.25 km and that this takes 2.8 hours.
If we consider the whole journey
and used the formula speed = total distance/total time we would get a speed of 188.25/2.8 =
67.23 kmhr-1. We call this value your average speed for the journey. It
does not mean that you travelled at this speed for the whole journey or even for very much of
it. (N.B multiplying your average speed by the total tim will give you the total distance
travelled =188.25 km).
(b) Instantaneous value of the quantity
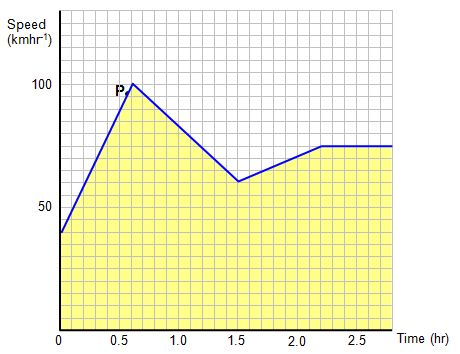
However, if at a
certain point on the journey you look at the speedometer and see that you are travelling at 96
kmhr-1 (60 mph). We call this value your instantaneous speed as it is
your speed at that particular instant (marked P on the graph). It would be found by taking
the short time (Dt) it takes the car to travel a short distance
(Ds). As before it does not mean that you travelled at this speed
for the whole journey or even for very much of it – it is simply your speed at that particular
moment.
Both these quantities can be considered using the following speed-time
graph.