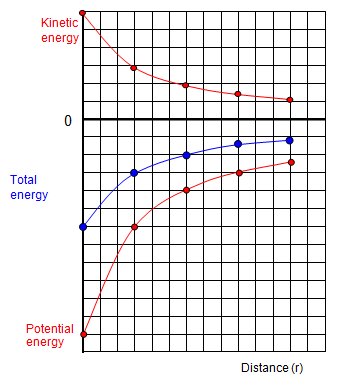
Gravitational energy graph for a satellite in orbit

The kinetic energy of an object is ½mv² and for a satellite in orbit this can be shown to be ½GMm/r where m is the mass of the satellite and r the radius of its orbit about a planet of mass M.
G is the constant of gravitation.

The gravitational potential energy of the satellite is - GMm/r

The total energy of the satellite -GMm/2r.

The variation of these three quantities with the radius of the orbit is shown in the following graph.










However if we consider a satellite on the surface of the Earth (radius R) then the total input of energy (E_L) required to put a satellite into an orbit of radius r is:

E_L = GMm/2r + [(- GMm/r) + (-GMm/R)] = GMm[1/R – 1/2r]

when r>R.

For the satellite to reach an infinite distance from the Earth the input of energy needed is GMm/R.