
Momentum ideas are also useful in explaining explosions. If we blow up a balloon and then burst it, the pieces of rubber fly off in all directions. The momentum of the balloon was zero before the explosion and therefore the total momentum of all the pieces of rubber after the explosion must also be zero. It is important to remember here that momentum is a vector and so the direction of motion of the pieces is important.
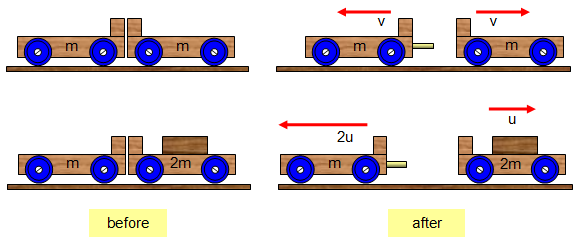
This principle can be studied with two spring-loaded trolleys. If they are put back to back and the spring in one is released they will explode. Both trolleys move apart with the same speed because they are of equal mass. In the second diagram trolley 2 moves off with half the speed of trolley 1 as it has twice the mass.
The recoil of a gun can be explained in the same way.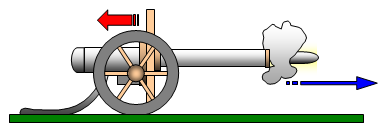
Before the gun is fired, the momentum is zero and so after firing the
momentum must still be zero, the momentum of the shell is equal and opposite to that of the
gun. A simple way of looking at it is this. If the shell is 100 times lighter than the gun then the
shell will move off 100 times faster than the gun recoils. Remember that in explosions the
lighter portion moves off faster than the heavier one.
