Astronomical distances

(GREEK MEASUREMENTS)

The size of the Earth

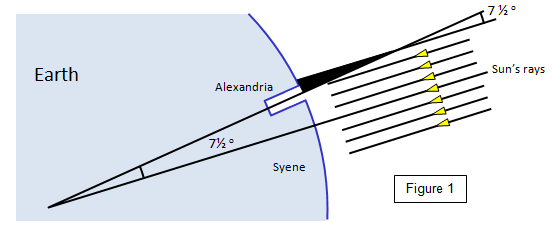
This was found in 240 BC by Eratosthenes. He found that the Sun's rays went vertically down a well at Syene at noon in mid summer, while they made an angle of 7½^o with a vertical stick at Alexandria, 800 km to the north.

You can see from Figure 1 that 7½^o is equivalent to 800 km of the Earth's circumference, and therefore the Earth has a circumference of 38 400 (24 000 miles). A very good result for those days.

The size and distance of the Moon

The size and distance of the Moon could be found once the size of the Earth was known. It was found that the Moon subtends an angle of when seen from the Earth, and so if we knew how big the Moon really was we could work out how far it is away from the Earth.

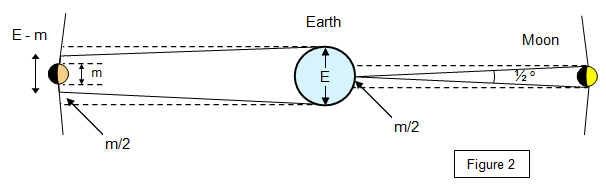
The Greeks noticed that in an eclipse of the Sun the Moon's shadow had narrowed to a point when it reached the Earth. They reasoned that the Earth's shadow would have narrowed by the same amount when it reached the Moon. So at the Moon the Earth's shadow would be E- m across (see Figure 2). In an eclipse of the Moon they found this shadow to be 2½ Moon diameters across and so the Earth is (2½ + 1) or 3½ times as big as the Moon. This gives the diameter of the Moon as roughly 3800 km.

Using the angular diameter of the Moon we can now work out how far it is from the Earth; it turns out to be about 250 000 miles. (400 000 km).

The size and distance of the Sun

Knowing how far away the Moon is can help in the measurement of the distance of the Sun. If you measure the angle between the Moon and the Sun at exactly half Moon then you have a diagram like the one below (Figure 3). You already know the distance of the Earth to the Moon and so knowing that the triangle is right-angled (half Moon) a measurement of the angle A will give you the distance of the Sun from the Earth using the formula:

cos A = Distance from the Earth to the Moon/Distance from the Earth to the Sun

The Greeks result was not very good as the angle is hard to measure. The actual value is very close to 90^o . (Angle A should be about 85.85^o). The distance obtained should be about 150 000000 km.

The angular size of the Sun is almost exactly the same as the Moon and so its actual size can be found as you now know the distance of the Sun from the Earth. Using this distance and the angular diameter of the Sun (0.5^o) gives the Sun a diameter of about 1400 000 km.

The Celestial Sphere

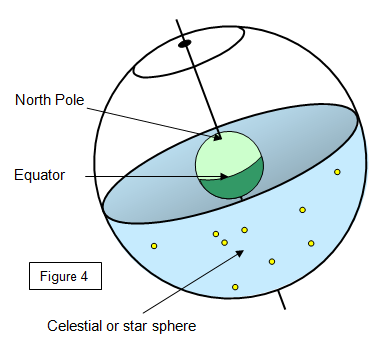
This diagram shows the Earth at the centre of the celestial sphere. The point where the projection of the polar axis of the Earth meets this sphere is called the celestial pole. A line drawn round the centre of the celestial sphere in the same plane as the equator of the Earth is called the celestial equator. As the Earth spins only the Pole Star seems to stay still, all the others appear to move round the Earth.

Circumpolar stars

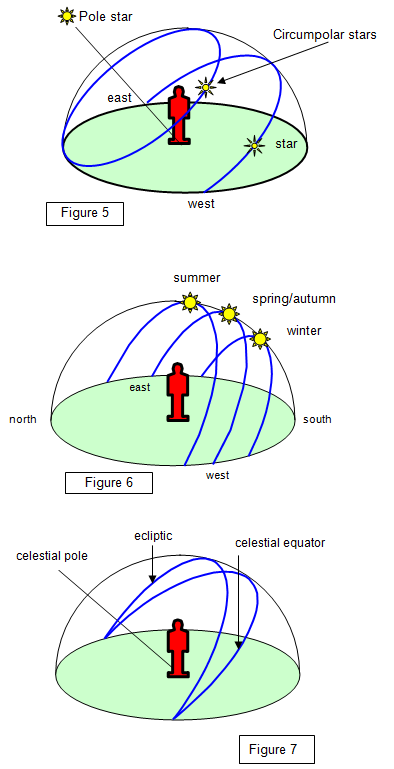
Some stars are far from the celestial pole and as the Earth rotates we see them rising in the east and setting in the west each night. However some stars are so close to the pole that they never set but can be seen every night of the year circling the pole star. These are known as circumpolar stars.

The Seasons and the motion of the stars

Because of the angle between the equator and the ecliptic the path of the Sun through the sky varies from one time of year to another. At the equinoxes it rises due east and sets due west but in summer and winter it follows a higher or lower path through the sky. (See figures 6 and 7)