E.m.f. generated in a rotating coil
Consider a coil of N
turns and area A being rotated at a constant angular velocity θ in a
magnetic field of flux density B, its axis being perpendicular to the field (Figure 1).
When the normal to the coil is at an angle θ to the field the flux through the coil is BAN
cosθ = BAN cos(ω)t, since θ = ωt.
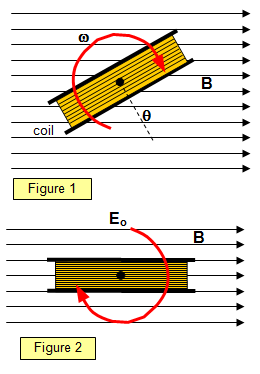
Therefore the e.m.f E generated between the ends of the coil
is:
E = -d(φ)/dt = -d(BANcosθ)/dt
Therefore:
E = BANωsinθ = BANωsin(ωt)
The
maximum value of the e.m.f (E
o) is when θ (= ωt) = 90
o (that is, the coil is in the plane of the field, Figure 2) and
is given by
Maximum e.m.f (Eo) = BANω
At this
point the wires of the coil are cutting through the flux at right angles – they chop through the field
lines rather than slide along them.
The r.m.s value of the e.m.f is (Er.m.s) = BANω/21/2

Coil positions and output voltage
Example problem
Example problem
Calculate the maximum value of the e.m.f generated in a coil with 200 turns and of area 10 cm2 rotating at 60 radians per second in a field of flux density 0.1 T.
E= BANω = 0.1x10-3 x 200 x 60 = 1.2 V
Notice the use of radians per second.
A VERSION IN WORD IS AVAILABLE ON THE SCHOOLPHYSICS USB