Stellar energy
It was soon realised that the energy of the stars was
produced by nuclear fusion, the temperatures required in the stars being lower than those
needed in the laboratory because of the enormous pressures in the stellar
interiors.
One such reaction that occurs in the Sun is the so called PP chain shown
below.
| 11H |
+ |
11H |
giving |
21H |
+ |
e+ |
+ |
energy |
| 21H |
+ |
11H |
giving |
32He |
+ |
energy |
| 32He |
+ |
32He |
giving |
42He |
+ |
211H |
+ |
energy |

Part of the energy in the first reaction is high-speed neutrinos and these can be detected on the Earth.
Note: the apparent imbalance
between particles in these equations can be understood if we look at the first of these. On
the left we have two protons and no neutrons. On the right we have one proton and one
neutron and a positron. One of the protons has been converted into a neutron and a
positron. Similar reasoning will explain the other equations.
Other reactions
may then occur such as:

Heavier and heavier nuclei can be produced by successive fusion reactions. The types of reaction taking place in the Sun are
shown below:
| 126C |
+ |
11H |
giving |
137N |
+ |
energy |
| |
|
137N |
giving |
136C |
+ |
e+ |
| 136C |
+ |
11H |
giving |
147N |
+ |
energy |
| 147N |
+ |
11H |
giving |
158O |
+ |
energy |
| |
|
158O |
giving |
157N |
+ |
e+ |
| 157N |
+ |
11H |
giving |
146C |
+ |
42He |
The net result of this
series of reactions is:
| |
411H |
giving |
442He |
+ |
2e+ |
energy |
In other words, 564 million
tonnes of hydrogen are being converted into 560 million tonnes of helium every second,
producing a staggering output of 3.90x10
20 MW - equivalent to some million million million
large power stations!
Although the Sun is converting four million tonnes of mass into
energy every second it is thought that it will continue to last for a further 10
9 years.
At higher temperatures (around 10
8 K) the fusion of helium starts the building of
heavier elements.
The reaction is:
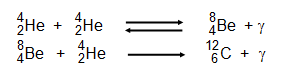
This is known as the
triple alpha process and is the first stage of 'helium burning'.
Beryllium 8 is very unstable
and so can revert to two alpha particles but eventually an equilibrium is set up and
maintained.




