

If you try to lift up a weight in a swimming pool and then try to lift
the same weight on the edge of the pool, it feels much lighter in the water.
This was
supposed to have been first explained by the Greek scientist Archimedes. He said that the
water gives an upward force or upthrust on any object in it.
You can weigh an
object in air and then in water and actually work out the upthrust, it is the difference between
the two readings. For this reason the upthrust is often called the loss in weight of the
object.
Archimedes principle states that:
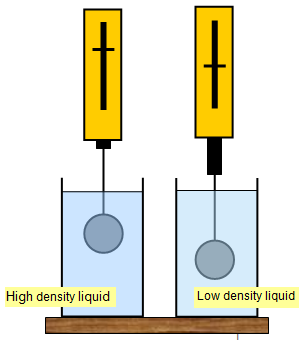
The weight of liquid displaced is the weight of the liquid that has been
replaced by the object. The volume of this amount of liquid is equal to the volume of the
object itself. The weight of fluid displaced and therefore the upthrust will be bigger if the
density of the liquid is large. The upthrust in salty water (relative density = 1.1) is larger than
that in water (relative density = 1.0) for the same object. This is why it is easier to swim in
the sea than in a freshwater lake.

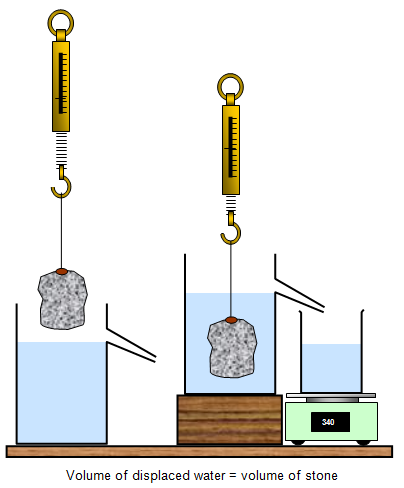
Archimedes principle says that when an object is immersed in a
liquid the apparent loss of weight of an object is equal to the upthrust and this is also equal
to the weight of the liquid displaced. You can prove this by the following
experiments.
Weigh an object in air and then lower it into a beaker of water that is
resting on a top pan balance. The reading on the spring balance will get less while the
reading on the top pan balance will increase by the same
amount.
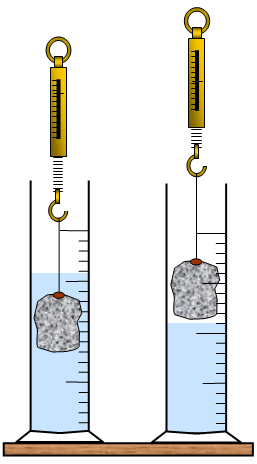
If you already know the density of the liquid
then you can simply measure the volume of displaced water and use mass = volume x
density to find its mass. This can easily be done using a measuring cylinder as shown in the
diagram.
You can see how the apparent weight of the
stone gets less when it is immersed in water. If it was only partly immersed it would appear
to weigh less than in air but not as little as when it is totally immersed in the
water.
A set of helium balloons fixed to a thin nylon thread makes an eye-catching display in a local car showroom. It is an excellent example of upthrust in a gas. What is the equation and name for the shape of the curve? Explain your answer.


